A GRAVITATIONAL
AND ELECTROMAGNETIC ANALOGY
BY OLIVER HEAVISIDE.
[Part I, The Electrician, 31, 281-282 (1893)]
(Here File for Word 97)
(Here web-page in other form (white BGCOLOR))
To form any notion at all of the flux of gravitational energy, we must
first localise the energy. In this respect it resembles the legendary hare
in the cookery book. Whether the notion will turn out to be a useful one
is a matter for subsequent discovery. For this, also, there is a well-known
gastronomical analogy.
Now, bearing in mind the successful manner in which Maxwell's localisation
of electric and magnetic energy in his ether lends itself to theoretical
reasoning, the suggestion is very natural that we should attempt to localise
gravitational energy in a similar manner, its density to depend upon the
square of the intensity of the force, especially because the law of the
inverse squares is involved throughout.
Certain portions of space are supposed to be occupied by matter, and
its amount is supposed to be invariable. Furthermore, it is assumed to
have personal identity, so that the position and motion of a definite particle
of matter are definite, at any rate relative to an assumed fixed space.
Matter is recognised by the property of inertia, whereby it tends to persist
in the state of motion it possesses; and any change in the motion is ascribed
to the action of force, of which the proper measure is, therefore, the
rate of change of quantity of motion, or momentum.
Let p be the density of matter, and e the intensity of force,
or the force per unit matter, then
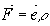 (1)
(1)
expresses the moving force on 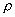 ,
which has its equivalent in increase of the momentum. There are so many
forces nowadays of a generalised nature, that perhaps the expression "moving
force" may be permitted for distinctness, although it may have been formerly
abused and afterwards tabooed.
,
which has its equivalent in increase of the momentum. There are so many
forces nowadays of a generalised nature, that perhaps the expression "moving
force" may be permitted for distinctness, although it may have been formerly
abused and afterwards tabooed.
Now the force 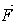 , or the
intensity
, or the
intensity 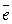 , may have many
origins, but the only one we are concerned with here is the gravitational
force. This appears to depend solely upon the distribution of the matter,
independently of other circumstances, and its operation is concisely expressed
by Newton's law, that there is a mutual attraction between any two particles
of matter, which varies as the product of their masses and inversely as
the square of their distance. Let
, may have many
origins, but the only one we are concerned with here is the gravitational
force. This appears to depend solely upon the distribution of the matter,
independently of other circumstances, and its operation is concisely expressed
by Newton's law, that there is a mutual attraction between any two particles
of matter, which varies as the product of their masses and inversely as
the square of their distance. Let 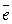 now
be the intensity of gravitational force, and
now
be the intensity of gravitational force, and 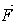 the
resultant moving force, due to all the matter. Then e is the space-variation
of a potential, say,
the
resultant moving force, due to all the matter. Then e is the space-variation
of a potential, say,
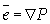 (2)
(2)
and the potential is found from the distribition of matter by
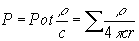 (3)
(3)
where 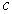 is a constant. This
implies that the speed of propagation of the gravitative influence is infinitely
great.
is a constant. This
implies that the speed of propagation of the gravitative influence is infinitely
great.
Now when matter is allowed to fall together from any configuration to
a closer one, the work done by the gravitational to reive is expressed
by the increase made in the quantity 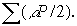 This
is identically the same as the quantity
This
is identically the same as the quantity 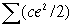 summed
through all space. If, for example, the matter be given initially in a
state of infinitely fine division, infinitely widely separated, then the
work done by the gravitational forcive in passing to any other configuration
is
summed
through all space. If, for example, the matter be given initially in a
state of infinitely fine division, infinitely widely separated, then the
work done by the gravitational forcive in passing to any other configuration
is 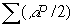 or
or 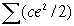 ,
which therefore expresses the "exhaustion of potential energy." We may
therefore assume that
,
which therefore expresses the "exhaustion of potential energy." We may
therefore assume that 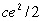 expresses
the exhaustion of potential energy per unit volume of the medium. The equivalent
of the exhaustion of potential energy is, of course, the gain of kinetic
energy, if no other forces have been in action.
expresses
the exhaustion of potential energy per unit volume of the medium. The equivalent
of the exhaustion of potential energy is, of course, the gain of kinetic
energy, if no other forces have been in action.
We can now express the flux of energy. We may compare the present problem
with that of the motion of electrification. If moved about slowly in a
dielectric, the electric force is appreciably the static distribution.
Nevertheless, the flux of energy depends upon the magnetic force as well.
It may, indeed, be represented in another way, without introducing the
magnetic force, but then the formula would not be sufficiently comprehensive
to suit other cases. Now what is there analogous to magnetic force in the
gravitational case? And if it have its analogue, what is there to correspond
with electric current? At first glance it might seem that the whole of
the magnetic side of elctromagnetism was absent in the gravitational I
analogy. But this is not true.
Thus, if u is the velocity of 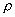 ,
then
,
then 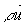 is the density of a
current (or flux) of matter. It is analogous to a convective current of
electrification. Also, when the matter enters any region through its boundary,
there is a simultaneous convergence of gravitational force into that region
proportional to
is the density of a
current (or flux) of matter. It is analogous to a convective current of
electrification. Also, when the matter enters any region through its boundary,
there is a simultaneous convergence of gravitational force into that region
proportional to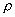 . This is
expressed by saying that if
. This is
expressed by saying that if
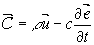 ,
(4)
,
(4)
then 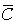 is a circuital flux.
It is the analogue of Maxwell's true current; for although Maxwell did
not include the convective term
is a circuital flux.
It is the analogue of Maxwell's true current; for although Maxwell did
not include the convective term 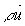 ,
yet it would be against his principles to ignore it. Being a circuital
flux, it is the curl of a vector, say
,
yet it would be against his principles to ignore it. Being a circuital
flux, it is the curl of a vector, say
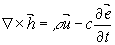 .
(5)
.
(5)
This defines 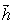 except as
regards its divergence, which is arbitrary, and may be made zero. Then
except as
regards its divergence, which is arbitrary, and may be made zero. Then 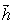 is
the analogue of magnetic force, for it bears the same relation to flux
of matter as magnetic force does to convective current. We have
is
the analogue of magnetic force, for it bears the same relation to flux
of matter as magnetic force does to convective current. We have
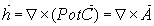 (6)
(6)
if 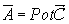 . But, since instantaneous
action is here involved, we may equally well take
. But, since instantaneous
action is here involved, we may equally well take
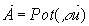 ,
(7)
,
(7)
and its curl will be 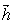 .
Thus, whilst the ordinary potential
.
Thus, whilst the ordinary potential 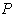 is
the potential of the matter, the new potential
is
the potential of the matter, the new potential 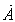 is
that of its flux.
is
that of its flux.
Now if we multiply (5) by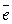 ,
we obtain
,
we obtain
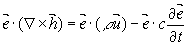 ,
(8)
,
(8)
or, which is the same,
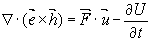 ,
(9)
,
(9)
if 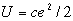 . But
. But 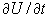 represents
the rate of exhaustion potential energy, so -
represents
the rate of exhaustion potential energy, so -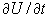 represents
its rate of increase, whiles
represents
its rate of increase, whiles 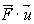 represents
the activity of the force on
represents
the activity of the force on 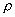 ,
increasing its kinetic energy. Consequently, the vector
,
increasing its kinetic energy. Consequently, the vector 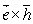 expresses
the flux of gravitational energy. More strictly, any circuital flux whatever
may be added. This
expresses
the flux of gravitational energy. More strictly, any circuital flux whatever
may be added. This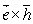 is analogous
to the electromagnetic
is analogous
to the electromagnetic 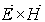 found
by Poynting and myself. But there is a reversal of direction. Thus, comparing
a single moving particle of matter with a similarly-moving electric charge,
describe a sphere round each. Let the direction of motion be the axis,
the positive pole being at the forward end. Then in the electrical case
the magnetic force follows the lines of latitude with positive rotation
about the axis, and the flux of energy coincides with the lines of longitude
from the negative pole to the positive. But in the gravitational case,
although h still follows the lines of latitude positively, yet since the
radial e is directed to instead of from the centre, the flux of energy
is along the lines of longitude from the positive pole to the negative.
This reversal arises from all matter being alike and attractive, whereas
like electrifications repel one another.
found
by Poynting and myself. But there is a reversal of direction. Thus, comparing
a single moving particle of matter with a similarly-moving electric charge,
describe a sphere round each. Let the direction of motion be the axis,
the positive pole being at the forward end. Then in the electrical case
the magnetic force follows the lines of latitude with positive rotation
about the axis, and the flux of energy coincides with the lines of longitude
from the negative pole to the positive. But in the gravitational case,
although h still follows the lines of latitude positively, yet since the
radial e is directed to instead of from the centre, the flux of energy
is along the lines of longitude from the positive pole to the negative.
This reversal arises from all matter being alike and attractive, whereas
like electrifications repel one another.
The electromagnetic analogy may be pushed further. It is as incredible
now as it was in Newton's time that gravitative influence can be exerted
without a medium; and, granting a medium, we may as well consider that
it propagates in time, although immensely fast. Suppose, then, instead
of instantaneous action, which involves
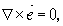 (10)
(10)
we assert that the gravitational force  in
ether is propagated at a single finite speed
in
ether is propagated at a single finite speed 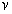 .
This requires that
.
This requires that
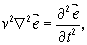 (11)
for this is the general characteristic of undissipated propagation at finite
speed. Now
(11)
for this is the general characteristic of undissipated propagation at finite
speed. Now

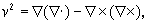
so in space free from matter we have
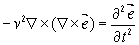 .
(12)
.
(12)
But we also have, by (5),
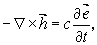 (13)
(13)
away from matter. This gives a second value to 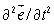 ,
when we differentiate (13) to the time, say
,
when we differentiate (13) to the time, say
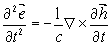 .
(14)
.
(14)
So, by (12) and (14), and remembering that we have already chosen 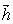 circuital,
we derive
circuital,
we derive
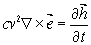 .
(15)
.
(15)
Or, if 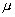 is a new constant,
such that
is a new constant,
such that
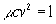 ,
(16)
,
(16)
then (15) may be written in the form
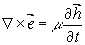 .
.
To sum up, the first circuital law (5), or
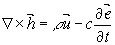
leads to a second one, namely (17), if we introduce the hypothesis propagation
at finite speed. This, of course, might be inferred from the electromagnetic
case.
In order that the speed 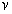 should
be not less than any value that may be settled upon as the least possible,
we have merely to make
should
be not less than any value that may be settled upon as the least possible,
we have merely to make 
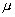 be
of the necessary smallness. The equation of activity becomes, instead of
(9),
be
of the necessary smallness. The equation of activity becomes, instead of
(9),
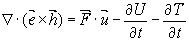 ,
(3)
,
(3)
if 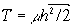 . The negative sign
before the time-increase of this quantity points to exhaustion of energy,
as before. If so, we should still represent the flux of energy by
. The negative sign
before the time-increase of this quantity points to exhaustion of energy,
as before. If so, we should still represent the flux of energy by 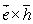 .
But, of course,
.
But, of course, 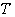 an almost
vanishing quantity when
an almost
vanishing quantity when 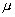 is
small enough, or
is
small enough, or 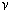 big enough.
Note that
big enough.
Note that 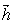 is not a negligible
quantity, though the product
is not a negligible
quantity, though the product 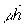 is.
Thus results will be sensibly as in the common theory of instantaneous
action, although expressed in terms of wave-propagation. Results showing
signs of wave-propagation would require an inordinately large velocity
of matter through the ether. It may be worth while to point out that the
lines of gravitational force connected with a particle of matter will no
longer converge to it uniformly from all directions when the velocity
is.
Thus results will be sensibly as in the common theory of instantaneous
action, although expressed in terms of wave-propagation. Results showing
signs of wave-propagation would require an inordinately large velocity
of matter through the ether. It may be worth while to point out that the
lines of gravitational force connected with a particle of matter will no
longer converge to it uniformly from all directions when the velocity 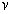 is
finite, but will show a tendency to lateral concentration, though only
to a sensible extent when the velocity of the matter is not an insensible
fraction of
is
finite, but will show a tendency to lateral concentration, though only
to a sensible extent when the velocity of the matter is not an insensible
fraction of 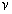 .
.
The gravitational-electromagnetic analogy may be further extended if
we allow that the ether which supports and propagates the gravitational
influence can have a translational motion of its own, thus carrying about
and distorting the lines of force. Making allowance for this convection
of  by the medium, with the
concomitant convection of
by the medium, with the
concomitant convection of 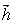 ,
requires us to turn the circuital laws (17), (18) to
,
requires us to turn the circuital laws (17), (18) to
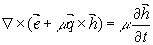 ,
(19)
,
(19)
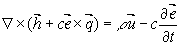 ,
(20)
,
(20)
where 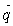 is the velocity
of the medium itself.
is the velocity
of the medium itself.
It is needless to go into detail, because the matter may be regarded
as a special and simplified case of my investigation of the forces in the
electromagnetic field, with changed meanings of the symbols. It is sufficient
to point out that the stress in the field now becomes prominent as a working
agent. It is of two sorts, one depending upon  and
the other upon
and
the other upon 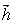 , analogous
to the electric and magnetic stresses. The one depending upon
, analogous
to the electric and magnetic stresses. The one depending upon 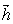 is,
of course, insignificant. The other consists of a pressure parallel to
is,
of course, insignificant. The other consists of a pressure parallel to 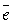 combined
with a lateral tension all round it, both of magnitude
combined
with a lateral tension all round it, both of magnitude 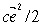 .
This was equivalently suggested by Maxwell. Thus two bodies which appear
to attract are pushed together. The case of two large parallel material
planes exhibits this in a marked manner, for e is very small between them,
and relatively large on their further sides.
.
This was equivalently suggested by Maxwell. Thus two bodies which appear
to attract are pushed together. The case of two large parallel material
planes exhibits this in a marked manner, for e is very small between them,
and relatively large on their further sides.
But the above analogy, though interesting in its way, and serving to
emphasise the non-necessity of the assumption of instantaneous or direct
action of matter upon matter, does not enlighten us in the least about
the ultimate nature of gravitational energy. It serves, in fact, to further
illustrate the mystery. For it must be confessed that the exhaustion of potential energy from a universal
medium is a very unintelligible and mysterious matter. When matter is infinitely
widely separated, and the forces are least, the potential energy is at
its greatest, and when the potential energy is most exhausted, the forces
are most energetic!
Now there is a magnetic problem in which we have a kind of similarity
of behaviour, viz., when currents in material circuits arc allowed to attract
one another. Let, for completeness, the initial state be one of infinitely
wide separation of infinitely small filamentary currents in closed circuits.
Then, on concentration to any other state, the work done by the attractive
forces it
represented by 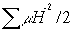 ,
where
,
where 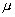 is the inductivity
and
is the inductivity
and 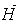 the magnetic force.
This has its equivalent in the energy of motion of the circuits, or may
be imagined to be so converted, or else wasted by friction, if we like.
But, over and above this energy, the same amount,
the magnetic force.
This has its equivalent in the energy of motion of the circuits, or may
be imagined to be so converted, or else wasted by friction, if we like.
But, over and above this energy, the same amount, 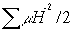 ,
represents the energy of the magnetic field, which can be got out of it
in work. It was zero at the beginning. Now, as Lord Kelvin showed, this
double work is accounted for by extra work in the batteries or other sources
required to maintain the currents constant. (I have omitted reference to
the waste of energy due to electrical resistance, to avoid complications.)
In the gravitational case there is a partial analogy, but the matter is
all along assumed to be incapable of variation, and not to require any
supply of energy to keep it constant. If we asserted that
,
represents the energy of the magnetic field, which can be got out of it
in work. It was zero at the beginning. Now, as Lord Kelvin showed, this
double work is accounted for by extra work in the batteries or other sources
required to maintain the currents constant. (I have omitted reference to
the waste of energy due to electrical resistance, to avoid complications.)
In the gravitational case there is a partial analogy, but the matter is
all along assumed to be incapable of variation, and not to require any
supply of energy to keep it constant. If we asserted that 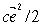 was
stored energy, then its double would be the work done per unit volume by
letting bodies attract from infinity, without any apparent source. But
it is merely the exhaustion of potential energy of unknown amount and distribution.
was
stored energy, then its double would be the work done per unit volume by
letting bodies attract from infinity, without any apparent source. But
it is merely the exhaustion of potential energy of unknown amount and distribution.
Potential energy, when regarded merely as expressive of the work that
can be done by forces depending upon configuration, does not admit of much
argument. It is little more than a mathematical idea, for there is scarcely
any physics in it. It explains nothing. But in the consideration of physics
in general, it is scarcely possible to avoid the idea that potential energy
should be capable of localisation equally as well as kinetic. That the
potential energy may be itself ultimately kinetic is a separate question.
Perhaps the best definition of the former is contained in these words:
- Potential energy is energy that is not known to be kinetic. But, however
this be, there is a practical distinction between them which it is found
useful to carry out. Now, when energy can be distinctly localised, its
flux can also be traced (subject to circuital indcterminatencss, however).
Also, this flux of energy forms a useful working idea when action at a
distance is denied (even though the speed of transmission be infinitely
great, or be assumed to be so). Any distinct and practical localisation
of energy is therefore a useful step, wholly apart from the debatable question
of the identity of energy advocated by Prof. Lodge.
From this point of view, then, we ought to localise gravitational energy
as a preliminary to a better understanding of that mysterious agency. It
cannot be said that the theory of the potential energy of gravitation exhausts
the subject. The flux of gravitational energy in the form above given is,
perhaps, somewhat more distinct, since it considers the flux only and the
changes in the amount localised, without any statement of the gross amount.
Perhaps the above analogy may be useful, and suggest something better.

